When comparing two quantities of the same kind, a ratio is utilized. For two numbers, a and b, the ratio formula is written as a: b or a/b. A ratio is said to be in proportion when two or more ratios are equal. Fractions are the foundation of the concepts of ratio and proportion. The fundamental building blocks for many other mathematical ideas are ratio and proportion. In many everyday situations, such as when comparing weights, heights, distances, or times, or when adding components to a recipe, ratios and proportions can be used to solve difficulties.
What is Ratio and Proportion?
A ratio is a comparison of two quantities by division, and a proportion is the equality of two ratios. A ratio is often expressed as x is to y, though it can also be written as x: y or x/y.
Conversely, proportion is an equation that asserts the equality of two ratios. A percentage can be read as x is to y as z is to w. It is written as x: y: z: w. In this case, where w and y are not equal to 0, x/y equals z/w.
Definition of Ratio
Ratio is the comparison of two quantities which is obtained by dividing the first quantity by the other. If a and b are two quantities of the same kind and with the same units, such that b is not equal to 0, then the quotient a/b is called the ratio between a and b. Ratios are expressed using the symbol of the colon (:). This means that ratio a/b has no unit and it can be written as a : b
Definition of Proportion
The equality of two ratios is referred to as proportion. There is always a proportionality between two equivalent ratios. The symbol (: 🙂 represents proportions, which aid in solving for unknown numbers. Put otherwise, a percentage is a formula or assertion that shows that two ratios or fractions are equal. If a: b = c: d, then the four non-zero quantities, a, b, c, and d, are said to be proportional. Let’s now examine the two ratios, 3: 5 and 15: 25. In this instance, 3:5 can be written as 3:5 = 3/5 = 0.6, and 15:25 as 15/25 = 3/5 = 0.6. We may claim that these two are proportional since they are both equal.
There are two types of proportions.
- Direct Proportion
- Inverse Proportion
Direct Proportion
Direct proportion describes the direct relationship between two quantities. If one quantity increases, the other quantity also increases and vice-versa. Thus, a direct proportion is written as y ∝ x. For example, if the speed of a car is increased, then it covers more distance in a fixed period of time.
Inverse Proportion
Inverse proportion describes the relationship between two quantities in which if one quantity increases, the other quantity decreases and vice-versa. Thus, an inverse proportion is written as y ∝ 1/x. For example, as the speed of a vehicle is increased, it will cover a fixed distance in less time.
Ratio and Proportion Formula
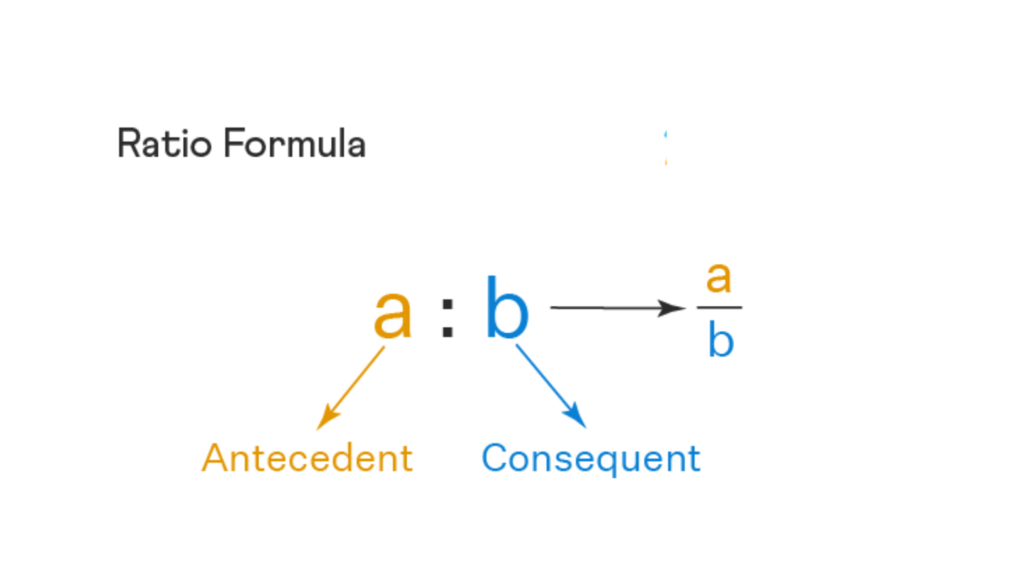
The formula for ratio is expressed as a : b ⇒ a/b, where,
- a = the first term or antecedent.
- b = the second term or consequent.
For example, ratio 2 : 7 is also represented as 2/7, where 2 is the antecedent and 7 is the consequent.
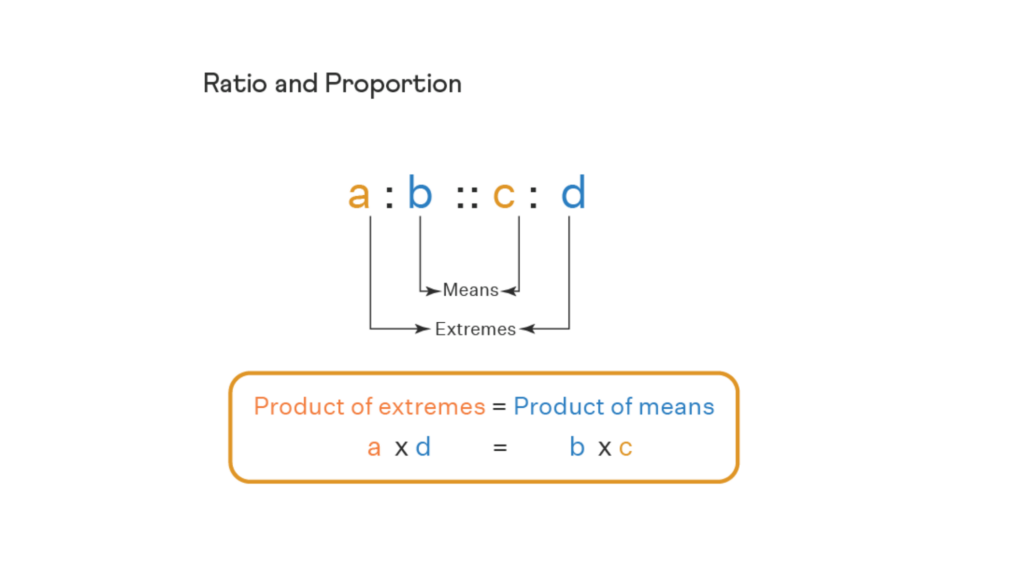
Now, in order to express a proportion for the two ratios, a : b and c : d, we write it as a:b::c:d⟶ab=cda:b::c:d⟶ab=cd
- B and C are referred to as mean words.
- Extreme terms are the two words a and d.
- While c and d may be independently of the same kind and of the same units, the values a and b in a: b = c: d should be of the same kind and have the same units. For instance, 5 kilogram x 15 kg = 75 x 225 rupees
- The formula for proportion can be written as follows: a/b = c/d or a: b: : c: d.
- The product of the extremes equals the product of the averages in a proportion. Consequently, we have b × c = a × d in the proportion formula a: b : c : d. For instance, we will have 15 × 75 = 5 × 225 in 5 : 15 :: 75 : 225.
Difference Between Ratio and Proportion
The difference between ratio and proportion can be seen in the following table.
| Ratio | Proportion |
|---|---|
| It is used to compare the size of two quantities with the same unit. | It is used to express the relation of two ratios. |
| The symbols used to express a ratio – a colon (:), slash (/) | The symbol used to express a proportion – double colon (::) |
| It is referred to as an expression. | It is referred to as an equation. |
Important Notes on Ratio and Proportion
- It is possible to compare any two quantities that have the same units.
- Only when two ratios are equal are they considered to be in proportion.
- The cross-product approach can also be used to determine whether two ratios are equal and proportionate.
- A ratio stays the same if we divide and multiply each of its terms by the same amount.
- Any three quantities are considered to be in a continuing proportion if the ratio of the first to the second is equal to the ratio of the second to the third.
- The ratio between the first and the second is equivalent to the ratio between the third and the fourth when any four quantities are in a continuous proportion.
It might be helpful